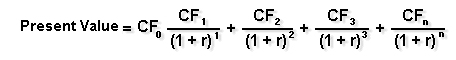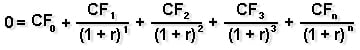Using ARR can give you a quick estimate of the project's net profits, and can provide a basis for comparing several different projects. Under this method of analysis, returns for the project's entire useful life are considered (unlike the payback period method, which considers only the period it takes to recoup the original investment). However, the ARR method uses income data rather than cash flow and it completely ignores the time value of money. To get around this problem, you should also consider the net present value of the project, as well as its internal rate of return.
Net present value of major purchases
The net present value method (NPV) of evaluating a major project allows you to consider the time value of money. Essentially, it helps you find the present value in "today's dollars" of the future net cash flow of a project. Then, you can compare that amount with the amount of money needed to implement the project.
If the NPV is greater than the cost, the project will be profitable for you (assuming, of course, that your estimated cash flow is reasonably close to reality). If you have more than one project on the table, you can compute the NPV of both, and choose the one with the greatest difference between NPV and cost.
As an example of how NPV works, imagine you're looking at a project costing $7,500 that is expected to return $2,000 per year for five years, or $10,000 in total. At first glance, the project looks profitable. Under the payback method, it looks as if the project will pay for itself in 3.75 years.
However, using NPV analysis, you can determine that if the discount rate on the project was 10 percent, the value of the expected returns would be $12,078.83. In other words, if you had $7,500.00 today and invested it at 10 percent, after five years you'd wind up with $12,078.83, well above your payback method calculation.
Bear in mind, though, that NPV analysis is generally used to evaluate the project's cash flows, rather than the income from the project that would be shown on an income statement. Why? Because the income statement factors in depreciation, but depreciation is not an out-of-pocket expense.
For instance, if revenue of $10,000 is reduced to $7,000 of income because of a $3,000 depreciation deduction, you still have the use of the full $10,000. So, the cash flow figure of $10,000 is the more instructive one to look at. However, if you are very concerned about the appearance of your income statement (for example, if you anticipate putting the business up for sale or seeking major financing in the future, or if you're under stockholder pressure to show more income) you may decide that the income figure is more appropriate to use.
How do you compute NPV? The easiest way is to use a good financial calculator. If you don't have one, or don't want to take the time to learn how to use one, you can use the present value table contained among the Tools and Forms.
Tools to use
Tools and forms contains a simple "present value of $1" table that you can use to figure the NPV of your project.
If you are mathematically inclined and have a calculator with exponential functions, you can also use the following formula:
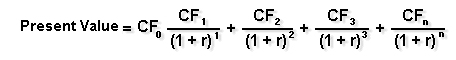
(When using this formula, CFx = cash flow in period x, n = the number of periods, and r = the discount rate.)
Whenever you do time value of money calculations to find a present or future value (such as NPV), you'll need to specify an interest rate, known as the discount rate. Choosing the appropriate discount rate is a very important part of the process.
Discount rate
How can you quickly estimate your cost of borrowing, which is used as the "discount rate," for purposes of analyzing a major purchase decision?
If you are planning to finance the purchase and you know what the interest rate on the loan would be, you can use the rate charged on the loan as the cost of borrowing for the project. Therefore, you would use the loan's rate as the "discount rate" in computing the net present value for the project. (If the rate is variable, you may have to take a guess as to the average rate over the loan period, or do the computation under worst-case and best-case scenarios.)
To fine-tune your calculations, you'll want to account for the fact that interest on business loans is tax-deductible. So, you can multiply the nominal interest rate on the loan by one minus the marginal tax rate for the business, to arrive at the tax-adjusted interest rate.
Example
If the rate on your loan was 8.5 percent and your marginal combined federal and state income tax rate was 40 percent, your tax-adjusted interest rate on the loan would be 5.1 percent (1- 0.40 = 0.60; 0.085 x 0.60 = 0.051).
If you are not financing your purchase, theoretically, you should attempt to compute an average cost of capital for your business that reflects all your current funding sources, including debt and owner's equity. Computing your true cost of capital can be rather time-consuming and complicated, and you'll probably need your accountant's assistance to do it accurately. The calculation depends on a number of economic conditions, opportunity costs, and business risks faced by the company.
What's more, using this figure assumes that additional capital can be obtained from similar sources in the same proportion, and at the same rates. For many small businesses, this may not be a realistic assumption. Instead, you can use your average cost of borrowing as the discount rate.
Example
The following figures reflect the cost to Clear Corporation of borrowing from each external source that it is currently using. We'll assume that Clear's common stock is currently not paying any dividends.